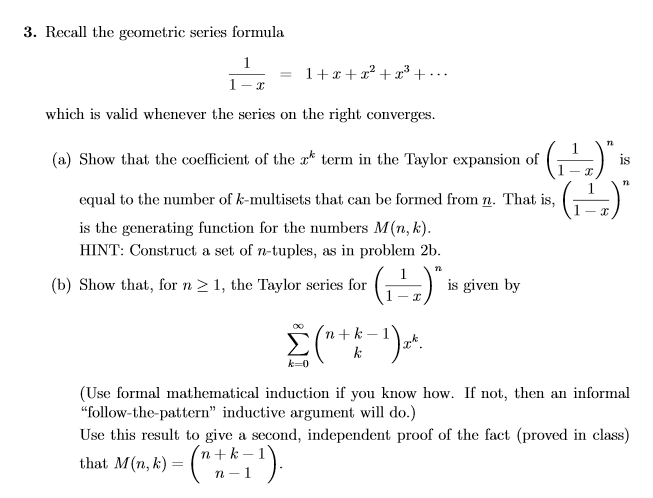
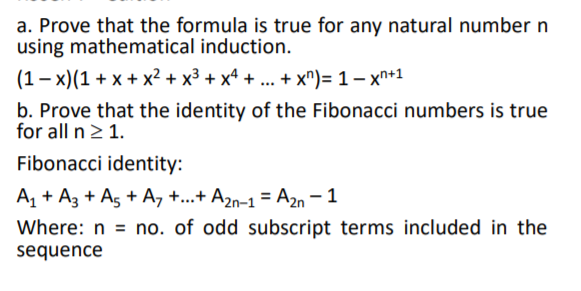
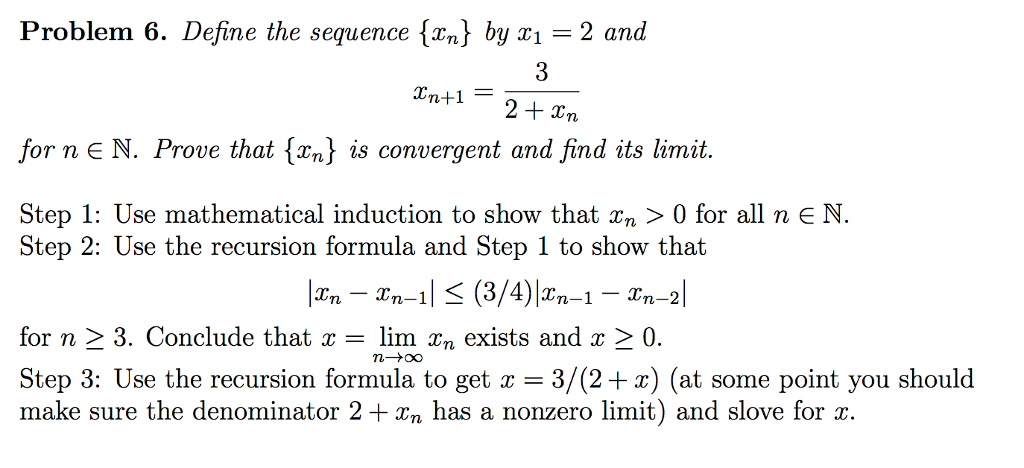
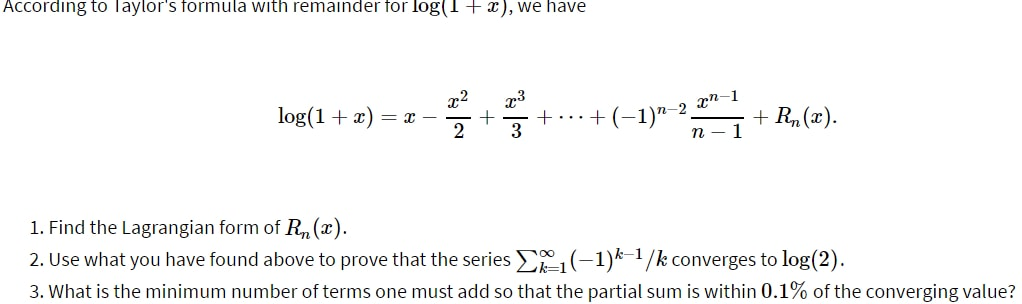1 X X 2 X 3 X N Formula Proof

Value of sum x n proof to the formula 1 x x 2 x 3 cdots x n frac x n 1 1 x 1 stack exchange network stack exchange network consists of 177 q a communities including stack overflow the largest most trusted online community for developers to learn share their knowledge and build their careers. Can anyone show me the proof of this equation. This is a mathematical series program where a user must enter the number of terms up to which the sum of the series is to be found.

gott srdce nehasnou hana hegerová matúš heger hasiči znojmo výjezdy hana svobodová instagram gratulace narozeninová přání přání k narozeninám gestační váček 5 týden těhotenství ultrazvuk heath ledger smrt harry styles concerto 2019

Don T Understand Why This Binomial Expansion Is Not Valid For X

Solved Recall The Geometric Series Formula 1 1 X 1 X X2

4 1 Higher Order Derivatives

What Is The Binomial Expansion For 1 X 1 Quora

Solved According To Taylor S Formula With Remainder For L

Binomial Theorem Properties Terms In Binomial Expansion

You may see this solution already f x 1 x x2 x3 x4 math f x 1 x x2 x3 x4 math f x 1 x 1 x x2 x3 math f x 1 x 1 x x2 x3 math f x 1 x f x.

1 x x 2 x 3 x n formula proof. Chapter 31 out of 37 from discrete mathematics for neophytes. Xn this is the finite geometric series because it has exactly n 1 terms. It has a simple formula. 1 x x 2.

Lim n to infty 1 x x 2 x 3 ldots x n frac 1 1 x where x 1. Pascal s ritangle the expansion of a x 2 is a x 2 a2 2ax x2 hence a x 3 a x a x 2 a x a2 2ax x2 a3 1 2 a 2x 2 1 ax x 3 a3 3a2x 3ax2 x urther f a x 4 a x a x 4 a x a3 3a2x 3ax2 x3 a4 1 3 a3x 3 3 a2x2 3 1 ax3 x4 a4 4a3x 6a2x2 4ax3 x4. Since the root of math x 1 math is math 1 math we simply have to check that math 1 math is a root of math x n 1 math. This is a geometric series with first term 1 and common ratio x.

The sum would be 1 x n 1 x. Number theory probability algorithms and other stuff by j. In general we see that the coe cients of a x n come from the n th row of pascal s. Take a number between 1 and 1 and raise it to a very high power and it goes to 0 we have the formula for the geometric series frac 1.

Lecture 27 11 7 power series 11 8 diﬀerentiation and integration of power series jiwen he 1 power series 1 1 geometric series and variations geometric series. Your series is a finite geometric series but without the first term. Following this we also need the value of x which forms the base of the series. Cargal 2 formula 1 the finite geometric series the finite geometric series the most basic geometric series is 1 x x2 x3 x4.

1x n 1 x 1 1 x n 1 x note. As n approaches infinity the sum approaches 1 1 x. If your series were. Note that a monomial is a factor of another monomial if they share the same roots.